Cell Adhesion Explained Through Many-Body Physics
Research news
Cells, the fundamental building blocks of all living organisms, stick to other cells via a process called cell adhesion. For multicellular organisms like ourselves, cell adhesion plays a crucial role in our immune response, in wound healing and cancer development, and it prevents us from degrading into a pool of individual cells. The last twenty years of experiments and theory have shown that cell adhesion may be accurately described by a model originally developed to describe ferromagnetism: the Ising model. Kristian Blom and Aljaž Godec at the Max Planck Institute for Biophysical Chemistry have now exploited this model to understand, through exact calculations, how the avidity of adhesion receptors and the strength of cellular adhesion can be regulated by mechanics. This led to some surprising results. (Physical Review X, September 27, 2021)
From ferromagnetism to cell adhesion
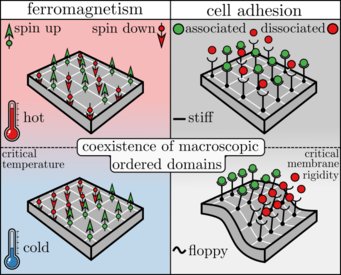
Ferromagnetism is the process through which certain materials spontaneously become magnetic when the temperature is lowered. This phenomenon can be explained by the Ising model, which was originally developed by the German Wilhelm Lenz in 1920. Each atom inside ferromagnetic material carries a magnetic dipole, here called a ’spin’, that can point in one of two directions: up or down. Spins interact with their direct neighbors via a coupling strength J, which favors the spins to align. At high temperatures the interaction has a weak effect (entropy dominates), and therefore on average half of the spins point up and the other half down, resulting in a vanishing net magnetic dipole. On the contrary, at small enough temperatures coupling dominates over entropy, resulting in clusters of spins aligned in the same direction, and thus a nonzero magnetic dipole. The temperature at which such spontaneous magnetization occurs is called the Curie or critical temperature.

Cell adhesion in turn arises through the association of cellular adhesion molecules (CAMs) which are attached to the cell membrane, or the cytoskeleton. CAMs can be either one of two states: associated or dissociated. The wiggling motion of the cell membrane as a result of thermal fluctuations gives rise to an interaction between nearby CAMs – CAMs tend to associate (dissociate) more easily if nearby CAMs are also associated (dissociated). This contributes to the so-called ‘avidity’ – N CAMs may collectively display a much stronger/weaker binding than N separate CAMs in solution.
The coupling decreases with increasing membrane rigidity: CAMs are non-interacting when embedded in very stiff membranes, and strongly interacting in floppier membranes (or cells). The membrane rigidity at which CAMs start to cluster or show ’collective behavior’ is called the ’critical membrane rigidity’, which relates directly to the Curie (or critical) temperature in ferromagnetism.
A new critical point in magnetism: the dynamical critical point
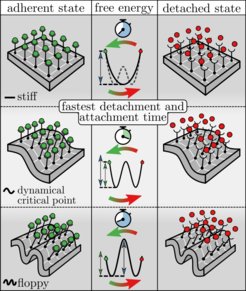
The strength of cell adhesion can be quantified experimentally by measuring the average detachment time of an adherent cell to a stiff substrate. With an atomic force microscope (AFM) an external force can be applied to the adherent cell, thereby driving the cell to the detached state. The stronger the adhesion strength, the longer it takes for a cell to detach from the substrate. It is intuitively expected that increasing the number of CAMs increases the adhesion strength and thus prolongs the detachment time. But what happens upon changing the membrane rigidity?
In their theoretical model, Blom and Godec have exactly calculated the average detachment time and its dependence on membrane rigidity. They did this for any number of CAMs, including the thermodynamic limit (of very many CAMs). What they found was surprising: a new critical (membrane) rigidity, coined the dynamical critical point, at which the average detachment time is minimal. Moreover, exactly at the dynamical critical point, average dissociation changes discontinuously with membrane rigidity – a hallmark of a critical point. The dynamical critical point is also present upon applying an external force to the cell, which is typically done in AFM experiments and therefore measurable experimentally.
The dynamical critical point provides a potential explanation for the experimentally observed softening of metastatic cancer cells that leave the initial tumor and spread to secondary locations in the body. Upon softening, membrane rigidity is speculated to approach the dynamical critical point. Therefore, the average detachment time from the primary tumor decreases, which in turn increases the metastatic potential of the cancer cell. Similarly, the result seems to have important implications for the immune response. Notably, cells can (and in fact do, according to experimental observations) actively tune membrane stiffness.
Interestingly, the fundamental principles underlying cell adhesion may be described by the same physics as magnetism and in turn, the same dynamical critical behavior is also implied for magnetization-reversal times.
In the future, Blom and Godec want to consider an even more detailed theoretical model of cell adhesion, which incorporates so-called catch bonds. This will hopefully lead to a deeper understanding of – and provide news insights into – cell adhesion under force. (kb/ag)